
Sapto Wahyu Indratno
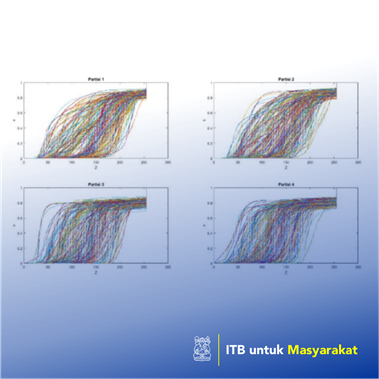
We applied Gaussian concept in image classification problem. For each partition we construct a cumulatif distribution function (CDF). Suppose we have M images and for each image we partition. Then for each partition we have M cumulatif distribution functions. We give an example of the M cumulatif distribution function on each partition. Here the M cumulatif distribution functions are the random sample, so we are interested in deriving a distribution of the CDF. Therefore we have to define a distribution of cumulatif distribution functions. Through this setting we hope that the huge information which contains in pixels can be replaced by just a distribution. In the next research we will define a test statistics which is needed in classifying an image into a class.
Penerapan Karya Tulis
A Gaussian Copula Process is a family of functions which satisfies the condition that at any finitely many points x1, x2, ... , xn the random vector (Φ−1(F1(f(x1))), Φ−1(F2(f(x2))), ... , Φ−1(Fn(f(xn))) is normally distributed with mean 0 and covariance Λ, where Fj(x) is the cumulative distribution function of random variable f(xj). In this approach Fj,j = 1,2, ... , n, are not necessarily normally distributed, therefore the Gaussian Copula Processes can be seen as generalization of Gaussian Processes.